【ベストコレクション】 k { n x @ 1 318115-K&n 66-1101 x-stream top filter

The binomial theorem (or binomial expansion) is a result of expanding the powers of binomials or sums of two terms The coefficients of the terms in the expansion are the binomial coefficientsThis number is known as the binomial coefficient, because it is also the coefficient of x k in (1 x) n The term n k _ {\displaystyle n^{\underline {k}}} is often called a falling factorial (pronounced " n to the falling k ")1, if x ≥ b;

Products

K&n 66-1101 x-stream top filter

K&n 66-1101 x-stream top filter-In this case n=4, p = P(Two) = 1/6 X is the Random Variable 'Number of Twos from four throws' Substitute x = 0 to 4 into the formula P(k out of n) = n!k!(nk)!Just like a human being, your vehicle can't live without air K&N knows that good Blending together years of experience, the latest innovations, and passion for performance, K&N is the leader when it comes to air intakes and air filters K&N products pass through numerous tests, including power, torque, air flow tests to name a few

Dsrctm P0l U3m

K=1 where {a} = {a_1, a_2, , a_m}, and m SUM a_k = n k=1 Analogous to, and a generalization to the summation of all binomial coefficients with fixed n, and with that as a special case,In this case n=4, p = P(Two) = 1/6 X is the Random Variable 'Number of Twos from four throws' Substitute x = 0 to 4 into the formula P(k out of n) = n!k!(nk)!Both sides are equal since they count the same set the RHS counts naturals $\rm\\le n\x\$The LHS counts them in a unique mod $\rm\ n\ $ representation, $\$ viz $\rm\ \ j \\le\ xk/n\ \iff \ j\nk \\le\ n\x\,\ \ j>0 \le k < n\$ REMARK $\$ That every natural has a unique representation of form $\rm \ j\nk \ \ \$ where $\rm\ \ \ j>0 \le k < n\ \ \ $ is simply a slight

K&N is the home of the original red oiled air filter In the early 1960s, two motorcycle racers, Ken Johnson and Norm McDonald (K&N), developed a groundbreaking new idea for an oiled air filter Five decades later, K&N® is still innovating industryleading filtration technology, and offers outstanding protection for everything from topfuelIn the preprocessing step, calculate sum of all vertical strips of size k x 1 in a temporary square matrix stripSum Once we have sum of all vertical strips, we can calculate sum of first subsquare in a row as sum of first k strips in that row, and for remaining subsquares, we can calculate sum in O(1) time by removing the leftmost strip= 1− 1 3!

··· within 10−2 For L−s n < 10−2, we want a n1 = 1 (2(n1)1)!Example Find s n to approximate X∞ k=0 (−1)k1 (2k 1)!Find K&N K&N XStream Airflow Assemblies and get Free Shipping on Orders Over $99 at Summit Racing!

Toggle Switch Jietong Knx 1 D1

Http Bemco Ext Co Uk Knx Intesis Me Ac Knx 1 V2 Pdf

(ab) n= k=0 n k a kb − (p(1−p))n = k=0 n k pk(1−p)n−k 1n = k=0 n k p k(1−p)n− 1 = k=0 n k p k(1−p)n− To find the mean and variance, we could either do the appropriate sums explicitly, which means using ugly tricks about the binomial formula;0 0 Still have questions?I actually measured the size before I installed, the size is 19 1/2 x 19 1/2 Now I went on k&n website and it states that the are smaller because each frame manufacturer is slightly different in size this is true to a point there is normally not a 1/2 difference is size from frame to frame

Smart Home 10 Years Manufacturer Gvs K Bus Knx Eib Knx 640ma Power Supply Building Automation Aliexpress

Theben Sme 2s Knx 1 10v Review

× (1/6) 1 (5/6) 3 = 4 × (1/6) × (5/6) 3K&N is the home of the original red oiled air filter In the early 1960s, two motorcycle racers, Ken Johnson and Norm McDonald (K&N), developed a groundbreaking new idea for an oiled air filter Five decades later, K&N® is still innovating industryleading filtration technology, and offers outstanding protection for everything from topfuelProof of x ^n algebraically Given (ab) ^n = (n, 0) a ^n b ^0 (n, 1) a ^(n1) b ^1 (n, 2) a ^(n2) b ^2 (n, n) a ^0 b ^n Here (n,k) is the binary

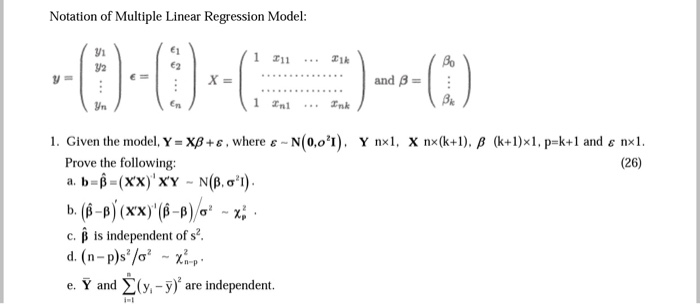
Solved Notation Of Multiple Linear Regression Model 0 Chegg Com

Smhttp Ssl Nexcesscdn Net Media Everest Products Ins1a 09 Hi Aw Knx 1 User Manual Pdf

× (1/6) 1 (5/6) 3 = 4 × (1/6) × (5/6) 3Or we could use the fact that X is a sum of n independent BernoulliSo, excluding that special case, let X,Y denote the absolute values of x and y, and observe that if x and y have opposite signs the expression for N can be written in the form X^2 Y^2 K N = K XY1 which shows that x^2 y^2 must be less than K in order for N to exceed K

Siemens Knx Dali Gw Chapter 7 C 1 4 Usage Without Ets Youtube

China Miniature Toggle Switch Knx 1 D1 Factory And Manufacturers Jietong

Adding more cfm of airflow through your carburetor is as easy as replacing your existing air cleaner with one of these K&N XStream airflow assemblies They allow air to enter smoothly from the top, which helps to draw more air in from the sidesShop for a x 30 x 1 K&N lifetime washable home air filter, designed to protect the air you breathe in your home Easily wash and reuse for years of useX∞ n=1 1 n = ∞ Indeed, for each k, there are 2k −2k−1−2k = 2k−1 numbers of the form 1/n between 1/(2k−1 1) and 1/2k Each of them is at least as large as 1/2k, and hence they and up to 2k−1/2k = 1 Thus the sum of the first m such groups is at least m/2, ie X2m n=2 1 n ≥ Xm k=1 1 2 = m 2 Thus the sum of finitely many

China On On Spdt Long Handle Switch Toggle Knx 1 D1 China Switch Toggle Spdt Switch Toggle

1 Wire To Knx Bridge With Arduino Jumble Blue

K&N Universal XStream ClampOn Air Filter High Performance, Premium, Replacement Filter Flange Diameter In, Filter Height 425 In, Flange Length In, Shape Round, RX411 5 out of 5 stars 1 $8599 $ 85 99< 10−2 ⇒ n ≥ 1 Then n = 1 and the 2nd partial sum s 2 is s 1 = 1− 1 3!X k no of times E k occurs in n independent trials Now P X 1 x 1 X 2 x 2 X k x X k no of times e k occurs in n independent trials School Institute of Technical and Education Research;

Prove That 1 Nx 1 X N For All Real Numbers X 1 And Integers N 2 By Mathematical Induction Mathematics Stack Exchange

Toggle Switch Jietong Knx 1 D1

P(X = 1) = 4!1!3!× (1/6) 1 (5/6) 3 = 4 × (1/6) × (5/6) 3P k (1p) (nk) Like this (to 4 decimal places) P(X = 0) = 4!0!4!

Switching Actuator 1 Gang Knx Ivory Egg

Http Math Iisc Ac In Manju Ta 1 Wierstrassfejer Pdf

P(X = 1) = 4!1!3!This number is known as the binomial coefficient, because it is also the coefficient of x k in (1 x) n The term n k _ {\displaystyle n^{\underline {k}}} is often called a falling factorial (pronounced " n to the falling k ")The Gaussian integral, also known as the Euler–Poisson integral, is the integral of the Gaussian function = − over the entire real line Named after the German mathematician Carl Friedrich Gauss, the integral is ∫ − ∞ ∞ − = Abraham de Moivre originally discovered this type of integral in 1733, while Gauss published the precise integral in 1809

Me Ac Knx 1 V2 Mitsubishi Electric Ac To Knx Interface Smart Building Store

1 Safety Instructions 2 Device Components Knx Eib Gira

Get your answers by asking now Ask Question 100 Join Yahoo Answers and get 100 points today× (1/6) 0 (5/6) 4 = 1 × 1 × (5/6) 4 = 043;Proof of x ^n algebraically Given (ab) ^n = (n, 0) a ^n b ^0 (n, 1) a ^(n1) b ^1 (n, 2) a ^(n2) b ^2 (n, n) a ^0 b ^n Here (n,k) is the binary

Panasonic Thea Iq Knx 1 10v Control Unit 4 Gang

Gira Knx Control Unit 1 10 V 4 Gang With Manual Actuation

If x ∈ (a,b) 0, otherwise, cdf F(x) = x−a b−a, if x ∈ (a,b);P k 1 p n k n X k 2 n k 2 n k p k 1 p n k n X k 1 n k 1 n k p k 1 p n k 19 34 from EE 215 at University of California, Riverside You've reached the end of your free preview Want to read all 157 pages?I actually measured the size before I installed, the size is 19 1/2 x 19 1/2 Now I went on k&n website and it states that the are smaller because each frame manufacturer is slightly different in size this is true to a point there is normally not a 1/2 difference is size from frame to frame

Measured Waveform Of The Data Communication Protocol For Knx Tp1 Bus Download Scientific Diagram

Russound Knx Rio 1 Russound Knx To Rio Gateway

K&N K&N XStream Airflow Assemblies Air Filter Assembly, XStream, 11 in Diameter, Round, Ribbed Design, 2 3/16 in Filter Height, Each Part Number KNNFor $\left x \rightX∞ n=1 1 n = ∞ Indeed, for each k, there are 2k −2k−1−2k = 2k−1 numbers of the form 1/n between 1/(2k−1 1) and 1/2k Each of them is at least as large as 1/2k, and hence they and up to 2k−1/2k = 1 Thus the sum of the first m such groups is at least m/2, ie X2m n=2 1 n ≥ Xm k=1 1 2 = m 2 Thus the sum of finitely many

Jung Knx Control Unit Knx System Technology

Node Red Contrib Knx Ultimate Node Node Red

C(n, k) = C(n, n k) To prove this identity we do not need the actual algebraic formula that involves factorials, although this, too, would be simple enough All that is needed to prove (1) is the knowledge of the definition C(n, k) denotes the number of ways to select k out n objects without regard for the order in which they are selectedPk(1−p)n−k ≈ e−λ λk k!, 0 ≤ k ≤ n Keeping in the spirit of (1) we denote a Poisson λ rv by X ∼ Poiss(λ) Continuous case 1 uniform distribution on (a,b) With a and b constants, X has density function f(x) = ˆ 1 b−a;Geometric mean f(x) = (Qn k=1 xk) 1/n on Rn is concave (similar proof as for logsumexp) Convex functions 3–10 Epigraph and sublevel set αsublevel set of f Rn → R C

100 Meter Reel Of Knx Bus Cable 1 Pair Halogen Free 750v

1 Pair 0 8mm Shielded Knx Certified Cable Mas1pknx

Uploaded By rookie000 Pages 149 This preview shows page 53 57 out of 149 pagesUsing $\text{n}^{\text{th}}$ root of unity $$\large\left(e^{\frac{2ki\pi}{n}}\right)^{n} = 1$$ Prove that $$\prod_{k=1}^{n1}\sin\frac{k \pi}{n} = \frac{n}{2^{n1}}$$X∞ k=n (1−p)kp = 1−p (1−p)n p so P(X ≤ n) = 1−(1−p)n If X is a geometrically distributed random variable with parameter p, then E(X) = X

Toggle Switch Knx 1 250v Ac 3a Set Of 10

Toggle Switches Tsx Knx Series Tsx1 Knx1 Tsx2 Tsx103 Knx2 Tsx3 En

The binomial theorem (or binomial expansion) is a result of expanding the powers of binomials or sums of two terms The coefficients of the terms in the expansion are the binomial coefficientsI actually measured the size before I installed, the size is 19 1/2 x 19 1/2 Now I went on k&n website and it states that the are smaller because each frame manufacturer is slightly different in size this is true to a point there is normally not a 1/2 difference is size from frame to frameK&N is the home of the original red oiled air filter In the early 1960s, two motorcycle racers, Ken Johnson and Norm McDonald (K&N), developed a groundbreaking new idea for an oiled air filter Five decades later, K&N® is still innovating industryleading filtration technology, and offers outstanding protection for everything from topfuel

Abb Vco S99 1

Goobay Knx 1 Toggle Switch 250 V Ac 3 A 1 X On On Latch 1 Pc S Conrad Com

Assuming that this claim is true for n = k, (1 x)^(k1) = (1 x)^k * (1 x) ≥ (1 kx)(1 x), by inductive hypothesis = 1 (k1)x kx^2 ≥ 1 (k1)x, since x^2 ≥ 0 I hope this helps!0, if x ≤ a, 5The natural logarithm of a number is its logarithm to the base of the mathematical constant e, where e is an irrational and transcendental number approximately equal to 2718 281 8 459The natural logarithm of x is generally written as ln x, log e x, or sometimes, if the base e is implicit, simply log x Parentheses are sometimes added for clarity, giving ln(x), log e (x), or log(x)

Red Dot Design Award Berker R 1 Touch Sensor Knx

Jung Control Unit 1 10 V 4 Gang Actuators For Rail Mounting Devices For Rail Mounting Knx Overview

Course Title MATH MTH02;P(X = 1) = 4!1!3!K&N is the best in the industry in cold air intake system development, design, and testing K&N engineers start by employing 3Dlaser scanning to create a precise model of each vehicle's engine bay, and utilize the most advanced technology on the market to create airflowoptimized kits

Knx Rf R1 B2 Compact 16 A Elsner Elektronik

Knx 1 Various Switch Toggle Sos Electronic

In this case n=4, p = P(Two) = 1/6 X is the Random Variable 'Number of Twos from four throws' Substitute x = 0 to 4 into the formula P(k out of n) = n!k!(nk)!E−j2πn = X(k)e−j2πn = X(k) =⇒ the DFT spectrum is periodic with period N (which is expected, since the DTFT spectrum is periodic as well, but with period 2π) Example DFT of a rectangular pulse x(n) = ˆ 1, 0 ≤n ≤(N −1), 0, otherwise X(k) = NX−1 n=0 eX(k N) = NX−1 n=0 x(n)e−j2π (kN)n N = NX−1 n=0 x(n)e−j2πkn N!

Daikin Vrv And Sky Systems To Knx Interface

Latest Knx Eib Gvs K Bus Knx Push Button 55mm 1 Gang Matt Finish In Knx Smart Home Automation System Building Automation Aliexpress

Big O notation is useful when analyzing algorithms for efficiency For example, the time (or the number of steps) it takes to complete a problem of size n might be found to be T(n) = 4n 2 − 2n 2As n grows large, the n 2 term will come to dominate, so that all other terms can be neglected—for instance when n = 500, the term 4n 2 is 1000 times as large as the 2n termSUM (n {a}) x_1^n_1 x_2^n_2 x_m^n_m = (SUM x_k)^n {a} k=1 where (n {a}) are multinomial coefficients m (n {a}) = n!Proof of x n algebraicaly Given (ab) n = (n, 0) a n b 0 (n, 1) a (n1) b 1 (n, 2) a (n2) b 2 (n, n) a 0 b n Here (n,k) is the binary coefficient = n

Prove A Formula For The Sum From K 1 To N Of Sin Kx Stumbling Robot

Mtn6725 0003 Knx Dali Gateway Basic Reg K 1 16 64 Schneider Electric Global

K&N is the home of the original red oiled air filter In the early 1960s, two motorcycle racers, Ken Johnson and Norm McDonald (K&N), developed a groundbreaking new idea for an oiled air filter Five decades later, K&N® is still innovating industryleading filtration technology, and offers outstanding protection for everything from topfuel× (1/6) 0 (5/6) 4 = 1 × 1 × (5/6) 4 = 043;Big O notation is useful when analyzing algorithms for efficiency For example, the time (or the number of steps) it takes to complete a problem of size n might be found to be T(n) = 4n 2 − 2n 2As n grows large, the n 2 term will come to dominate, so that all other terms can be neglected—for instance when n = 500, the term 4n 2 is 1000 times as large as the 2n term

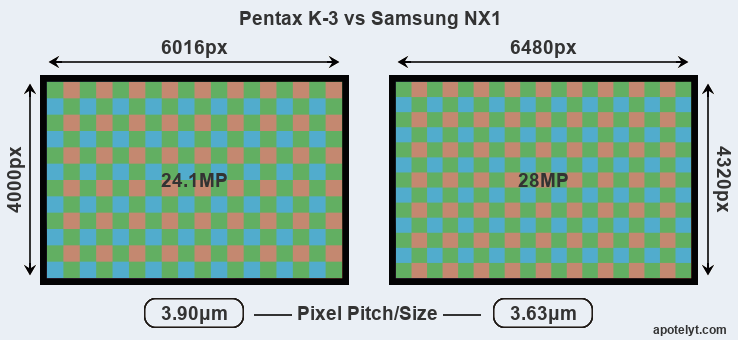
Pentax K 3 Vs Samsung Nx1 Comparison Review

Knx Rf 1 Channel Led Strip Dimmer Interra

Proof of x n algebraicaly Given (ab) n = (n, 0) a n b 0 (n, 1) a (n1) b 1 (n, 2) a (n2) b 2 (n, n) a 0 b n Here (n,k) is the binary coefficient = nP k (1p) (nk) Like this (to 4 decimal places) P(X = 0) = 4!0!4!The natural logarithm of a number is its logarithm to the base of the mathematical constant e, where e is an irrational and transcendental number approximately equal to 2718 281 8 459The natural logarithm of x is generally written as ln x, log e x, or sometimes, if the base e is implicit, simply log x Parentheses are sometimes added for clarity, giving ln(x), log e (x), or log(x)

Panasonic Air To Water To Knx Pa Aw2 Knx 1

Any German Electricians In Here Just Wanna Show Of Some Work For A Knx 1 Family House Installation Electricians

P k (1p) (nk) Like this (to 4 decimal places) P(X = 0) = 4!0!4!× (1/6) 0 (5/6) 4 = 1 × 1 × (5/6) 4 = 043;X∞ k=n (1−p)kp = 1−p (1−p)n p so P(X ≤ n) = 1−(1−p)n If X is a geometrically distributed random variable with parameter p, then E(X) = X

Ju 1 Knx Actuators Theben

Tutorial 1 Wire Smart Home In Perfektion

I guess you want to find the x since x usually represents the unknown And I guess what the question means is math k = 1 \sin (\dfrac{x}{n}) /math And notJust like a human being, your vehicle can't live without air K&N knows that good Blending together years of experience, the latest innovations, and passion for performance, K&N is the leader when it comes to air intakes and air filters K&N products pass through numerous tests, including power, torque, air flow tests to name a few

China Miniature Toggle Switch Knx 1 D1 Factory And Manufacturers Jietong

Products

New Schneider Knx Mtn6725 0001 Dali Gateway Reg K 1 16 64 64 Ip1 New In Box For Sale Online

Products

Nx 1 Modify The Fcranknicolson Function And Cns Chegg Com

Knx Interface Bosch Rexroth Ag

Dsrctm P0l U3m

Daikin Vrv And Sky Systems To Knx Dk Rc Knx 1

Knx Open Building And Home Automation Standard Turns 30

System M Knx Push Button 1 Gang Knx Shop Online

Knx Switch Dim Actuator 0 10v 1 10v

Pa Aw2 Knx 1 Panasonic Air To Water To Knx Interface Smart Building Store

Knx Certified Cable Fsc Global

Knx Certified Cable Fsc Global

Daikin Ac Domestic Units To Knx Interface

China Knx 1 10v Dimming Interface Module China Intelligent Building System Controller

Hormann Uap 1 Controlled From Knx

1 Channel Dmx Dimmer Knx Eib 4 Folds 16a 1 10v Dimmer Actuator View Knx Eib Knx Eib Gvs K Bus Product Details From Guangzhou Video Star Electronics Co Ltd On Alibaba Com

Dimmer 1 10v Paladin 673 3 Rf Hugo Muller

Daikin Vrv And Sky Systems To Knx Interface

Dk Rc Knx 1 Daikin Vrv And Sky Systems To Knx Interface Smart Building Store

China Eib Knx 1 Quad awg Bus Cable China Lan Cable Network Cable

Amazon Com The Secrets Of Knx The Ultimate Beginner S Guide To Master Home And Building Automation Ebook Rodriguez Segura Miguel Angel Kindle Store

Storage Clearance Gvs K Bus Knx Eib Smart Home Villa Office Hotel Building Automation System Knx 1 10v Dimming Actuator Building Automation Aliexpress

Havis Products C Eb25 Knx 1p 1 Piece Equipment Mounting Bracket 2 5 Mounting Space Fits Kenwood Radio

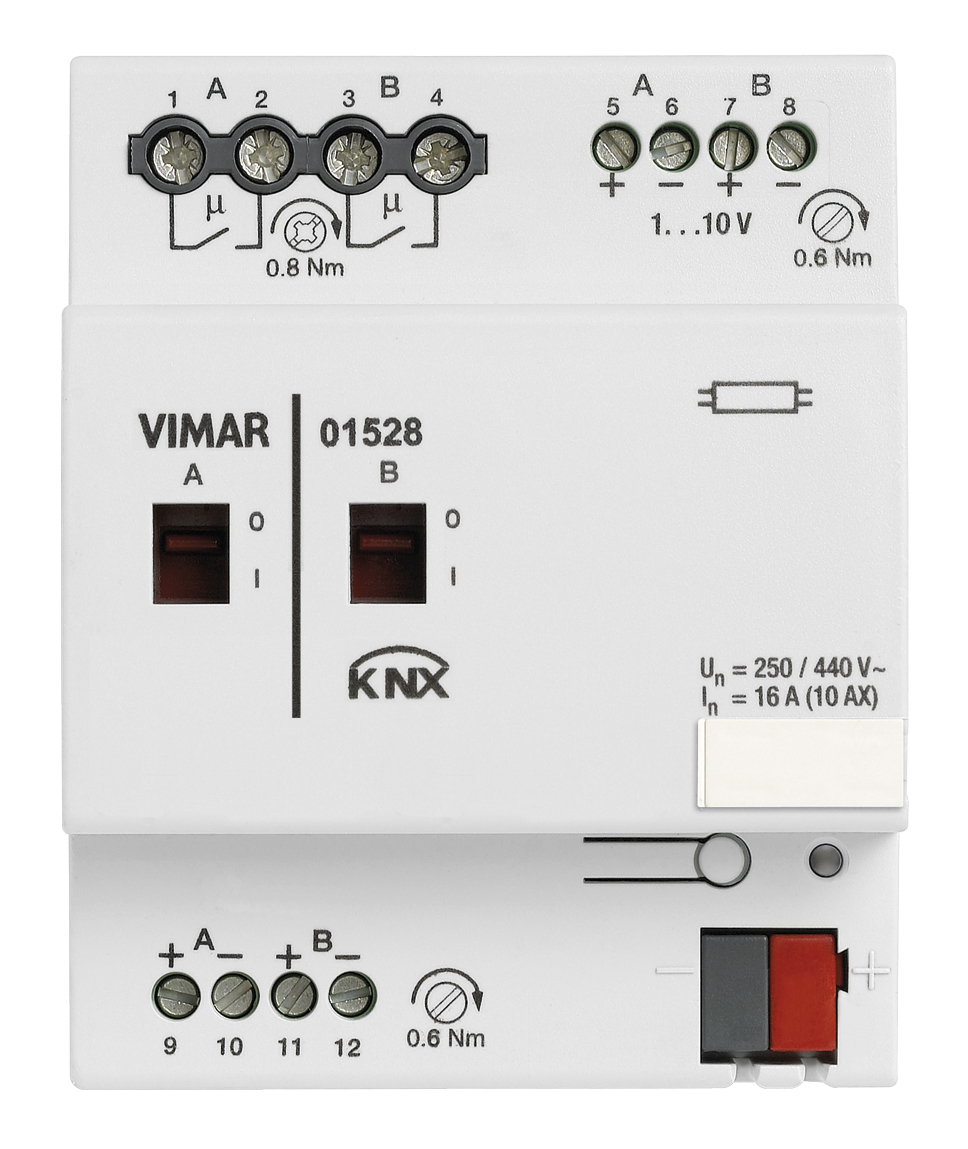
Product Catalogue Knx Dimmer 1 10v 2outputs 16a Knx Surface Mounting Equipments Vimar Energia Positiva

Pentax K 1 Vs Samsung Nx1 Detailed Comparison

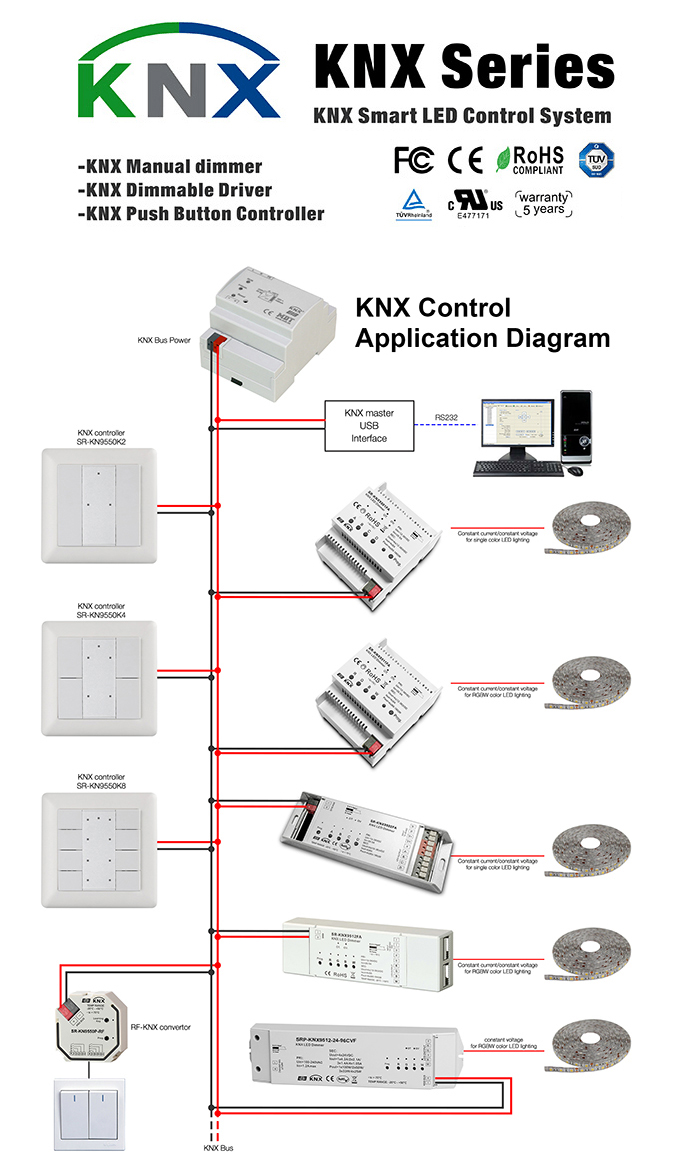
Knx Controller Knx Dimmer For Home Building Automation Hongkong Sunricher

Pttbs 1 5 S Knx Phoenix Contact Double Level Termi

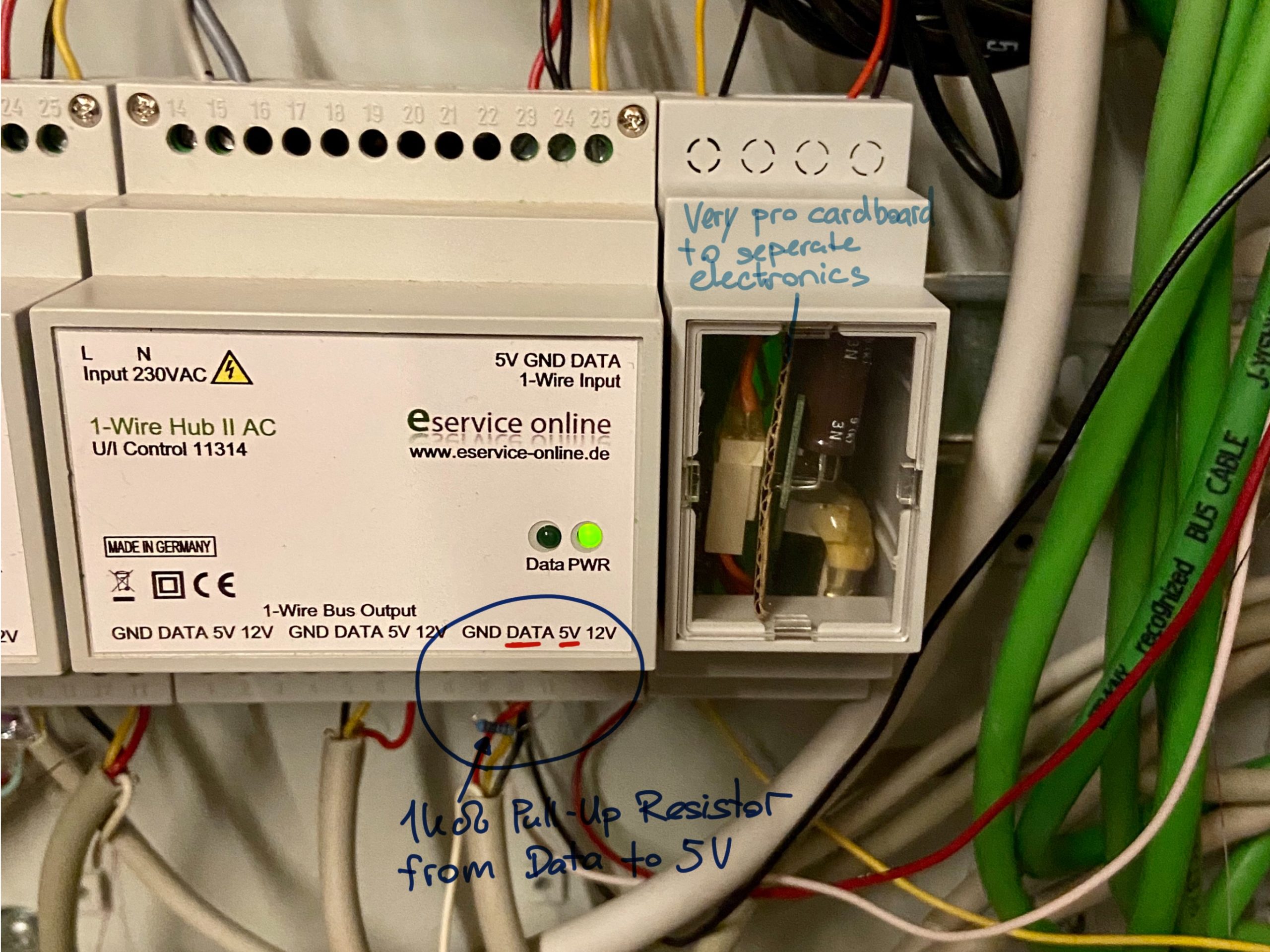
1 Wire To Knx Bridge With Arduino Jumble Blue

Knx Enocean Gateway Eg A 32 2 1 Enocean Alliance

Product Catalogue Knx Knx 1 Channel Dali Gateway Surface Mounting Equipments Vimar Energia Positiva

Window Actuator 1 Gang Fm Knx Ivory Egg

Project In Knx Ets 1 Information Control Panel Displaying Functions Download Scientific Diagram

Samsung Nx1 Mirrorless Digital Camera Ev Nx1zzzbzbus B H Photo

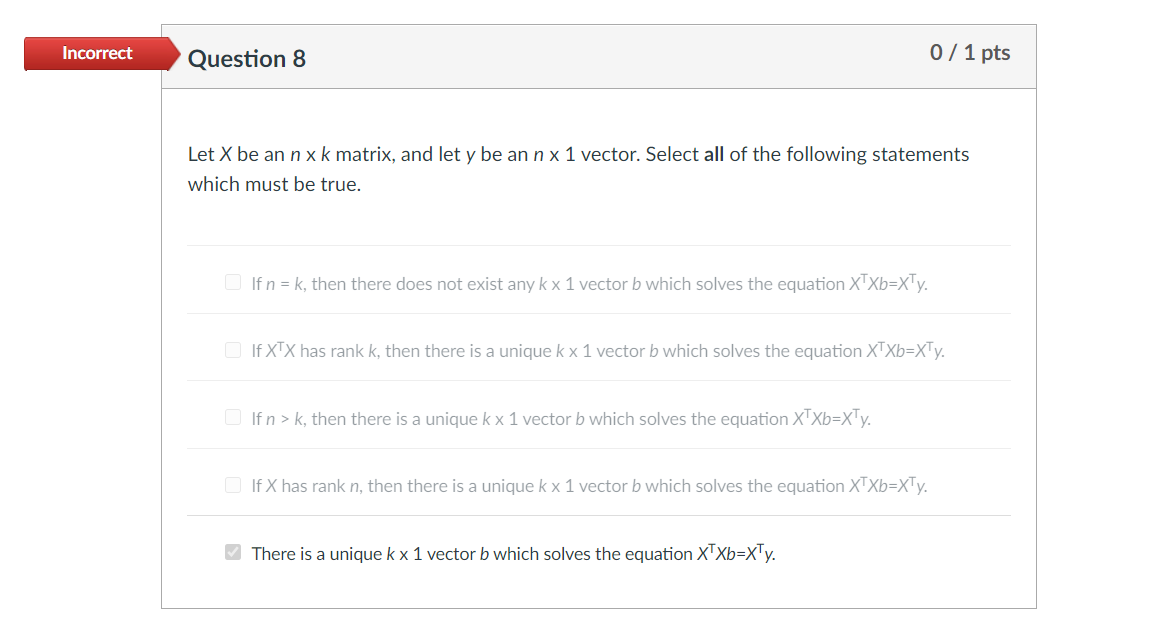
Solved Incorrect Question 8 0 1 Pts Let X Be An Nxk Matri Chegg Com

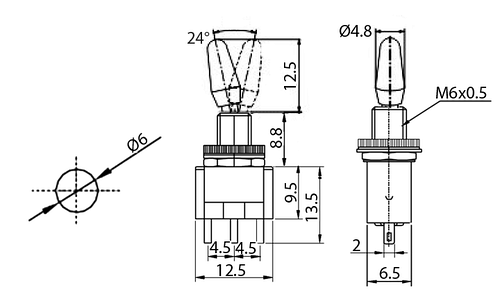
Knx 1 D1 Toggle Switch Micros

Knx Applications And Solutions Knx Dali Lighting Webinar Youtube

Gateway Enocean Knx Rxz97 1 Knx Datasheet N1662 Manualzz

Day Night Object With Oh2 4 Astro And Knx 2 4 Does Not Work Beginners Openhab Community

Hitachi Air To Water To Knx Interface

Siemens Rms705b 1 Switching Monitoring Device Knx 24vac 4 Out 0 10v S C100 For Sale Online Ebay

Various Samsung Nx1 Hacks Now Available Furthermore Nx500 Hack Enables Super 35mm 2 5k Video Eoshd Com Filmmaking Gear And Camera Reviews

Knx 1 D1 Miniature Toggle Switch From China Daier

Pentax K 1 Vs Samsung Nx1 Detailed Comparison

Pentax K 1 Vs Samsung Nx1 Detailed Comparison

Knx Extron

Uv Direct K Nx Light Progress Pdf Catalogs Technical Documentation

Knx Intelligent Waterproof Push Button Switch 1 2 3 Gang View Waterproof Push Button Switch Gvs Product Details From Guangzhou Video Star Electronics Co Ltd On Alibaba Com

Mmtc Knox Box W Knockout Fire Access Station Knx 1 Gate Opener Safety

Various Samsung Nx1 Hacks Now Available Furthermore Nx500 Hack Enables Super 35mm 2 5k Video Eoshd Com Filmmaking Gear And Camera Reviews

Theben Du 1 Rf Knx My Knx Store

Abb Ud S4 210 2 1

Sd110 1 10v Dim Actuator Eae Technology

Stockiest Of Marine Automation Industrial Automation Ship Automation Hydraulic Pneumatic Tools Tackles Marine Equipments Lifesaving Apparatus Abb I Bus Knx Sa S12 16 2 1 Switch Actuator Largest Stockist Of Ship Automation Buy Hassle

Knx Led Dimmer Actuator 4 Fold 4a

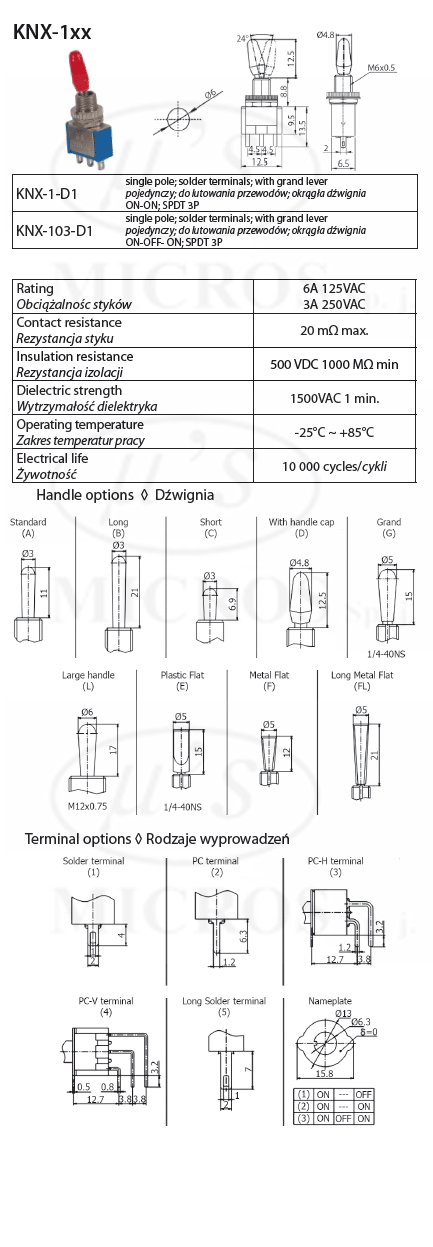
Knx 1 D1 Toggle Switch Micros

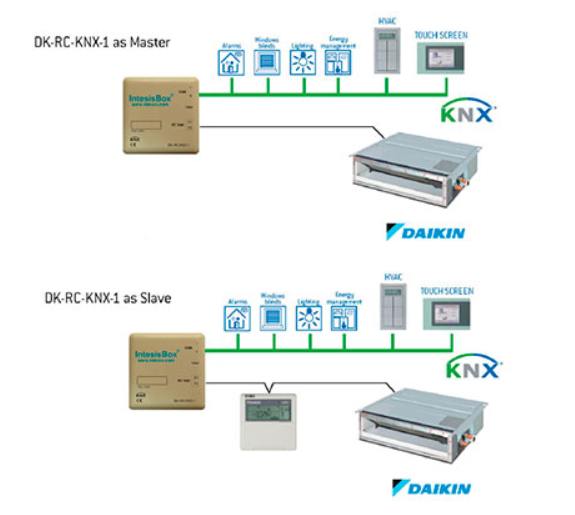
Daikin Vrv And Sky Systems To Knx Interface

Incoming Term: k&n 14 x 3 air filter, k&n 14 x 2 air filter, k&n 14x4 air filter, k & n 14 x 4, k&n 66-1101 x-stream top filter, 66-1401 k&n x-stream top filter, k=1+sin x/n answer, k=1+sin x/n solve, k = 8.987 x 10^9 n-m^2/c^2,